Tanya Khovanova's Math Blog

I recently received an invoice from Jumpline, Inc. requesting a payment for hosting www.tanya-khovanova-temp.com. I had never heard of Jumpline before and I didn’t have a webpage with that address. So I thought that it was spam.

Because the invoice had my name and address, I decided to call them and check what was going on. It appeared that Jumpline had swallowed Hosting Rails, the company that was hosting my Number Gossip page. Still, I didn’t have a clue what the invoice was about.

I asked the representative whether the web address was related to Number Gossip, and he said no. So I canceled the hosting. My work schedule is the busiest in July, so I forgot about the invoice and didn’t check my website.

Then I received a letter from Christian, a Number Gossip fan, who told me that the website was down. I called Jumpline again.

It appears that the representative didn’t know what he was doing and misled me. The web address www.tanya-khovanova-temp.com was an internal name for my Number Gossip site. They had deleted all the files and were unable to restore my website.

Now I have to decide what to do. I do not want to go back to Jumpline as they are very unprofessional in these ways:

• hey didn’t notify me that Hostingrails.com no longer exists.
• They didn’t give me a new password that would have allowed me to look at my account. I had to do everything by phone.
• They sent me a confusing invoice that I was certain not to recognize.
• Their representative didn’t have a clue what was going on.
• They couldn’t restore the webpage I had for years although they had only canceled it less than two weeks before.
• The representative promised to connect me to a manager and hung up.

Can anyone suggest a company that can host a website that is written in Ruby on Rails?

The Fibonacci sequence is all about addition, right? Indeed, every element F_n of the Fibonacci sequence is the sum of the two previous elements: F_n = F_n-1 + F_n-2. Looking closer we see that the Fibonacci sequence grows like a geometric progression φⁿ, where φ is the golden ratio. In addition, the Fibonacci sequence is a divisibility sequence. Namely, if m divides n, then F_m divides F_n.

My point: we define the sequence through addition, and then multiplication magically appears by itself. What would happen if we tweak the rule and combine addition and multiplication there?

John Conway did just that: namely, he invented a new sequence, or more precisely a series of sequences depending on the pair of the starting numbers. The sequences are called Conway’s subprime Fibonacci sequences. The rule is: the next term is the sum of the two previous terms, and, if the sum is composite, it is divided by its least prime factor.

Let me illustrate what is going on. First we start with two integers. Let’s take 1 and 1 as in the Fibonacci sequence. Then the next term is 2, and because it is prime and we do not divide by anything. The next two terms are 3 and 5. After that the sum of two terms is 8, which is now composite and it is divided by 2. So the sequence goes: 1, 1, 2, 3, 5, 4, 3, 7, 5, 6, 11 and so on.

The subprime Fibonacci sequences excite me very much. Not only does adding some multiplication to the rule make sense to me, but also, the sequences are fun to play with. I got so excited that I even coauthored a paper about these sequences titled, not surprisingly, Conway’s Subprime Fibonacci Sequences. The paper is written jointly with Richard K. Guy and Julian Salazar, and is available at the arXiv:1207.5099.

We can start a subprime Fibonacci sequence with any two positive numbers. You can see that such a sequence doesn’t grow fast, because we divide the terms too often. We present a heuristic argument in the paper that allows us to conjecture that no subprime Fibonacci sequence grows indefinitely, but they all start cycling. The conjecture is not proven and I dare you to try.

Meanwhile, the sequences are a lot of fun and I suggest a couple of exercises for you:

• Prove that there are no cycles of length two or three.
• Prove that the maximum number in a non-trivial cycle is prime.
• Prove that the smallest number in a non-trivial cycle is more than one. You can prove that it is more than 6 for extra credit.

By the way, a trivial cycle is the boring thing that happens if we start a sequence with two identical numbers n bigger than one: n, n, n, n, ….

Have fun.

Kvant is a very popular Russian math and physics journal for high school-children. My favorite page is the one with puzzles directed to younger readers. Here are two puzzles from the latest online issue: 2012 number 3.

The first one, by N. Netrusova, is optimistic about the next year.

An astrologist believes that a year is happy if its digit representation contains four consecutive digits. For example, the next year, 2013, will be happy. When was the previous happy year?

The second problem is by L. Mednikov and A. Shapovalov. It confused me at first. For a moment I thought that the best answer is 241 rubles:

A big candle lasts one hour and costs 60 rubles. A small candle lasts 11 minutes and costs 11 rubles. Can you measure a minute by spending not more than a) 200 rubles, b) 150 rubles?

My American friends often ask me for insights into why Grigory Perelman refused the one million dollar Clay prize for his proof of the Poincaré conjecture. They are right to ask me: my life experience was very similar to Perelman’s.

I went to a high school for children gifted in math. I was extremely successful in competitions. I got my gold medal at IMO and went to college without entrance exams. I received my undergraduate and graduate degrees in one of the best math academic centers in Soviet Russia. Perelman traveled a similar path.

Without ever having met Perelman, I can suggest two explanations of why he might reject the money.

First explanation. To have it publicly known that you have suddenly come into money is very dangerous in Russia. Perelman’s life expectancy would have dropped immediately after accepting the million dollars. Russians that have tons of money either hide their wealth or build steel doors way before they make their first million. In addition to being a life hazard, money attracts a lot of bother. He would have been chased by all types of acquaintances asking for help or suggesting marriage proposals.

Second explanation. We grew up in a communist culture where money was scorned and math was idolized. The goal of research was research. Proving the conjecture was the prize itself. In his mind, receiving the award money might diminish the value of what he did. I understand this way of thinking, but I am personally too practical to follow such feelings and would accept the prize.

My first explanation has a flaw. Though valid, it doesn’t explain why he rejected the Fields medal. So I reached for the book abour Perelman, Perfect Rigor: A Genius and the Mathematical Breakthrough of the Century by Masha Gessen. I like Gessen’s explanation of why he rejected the Fields medal:

His objection to the Fields Medal, though never stated as clearly, seemed to have been twofold: first: he no longer considered himself a mathematician and hence could not accept a price intended for the encouragement of midcareer researchers; and second, he wanted no part of ICM, with all the attendant publicity, speeches, ceremony, and king of Spain.

The reasons are specifically related to the medal, so the Clay prize rejection might not be connected to the medal rejection. This argument slightly rehabilitates my first explanation.

I liked the book. It is a tremendous undertaking — writing about a person who doesn’t want to talk to anyone. After reading it, I have one more possible explanation of his refusal of the prize.

Perelman is a loner. One of the closest people to him was his math Olympiad coach. The coaches tend to understand the solutions on the spot, mostly because they already know them. If in his mind Perelman expected all mathematicians to be like his coach, then he might have expected a parade in his honor the day after he solved the conjecture. Instead, he got silence and attempts to steal the prize from him.

Can you imagine doing the century’s best math work without receiving congratulations for many years? The majority of mathematicians waited for the judgment of the experts, as did Perelman. The experts were busy and much slower than Perelman expected. The conjecture was extremely difficult, and it was a high-profile situation — after all, $1 million was attached to its solution. So the experts were very cautious in their pronouncements.

Finally, instead of congratulating Grigory, they said that the proof seemed to be correct and that they had not yet found any mistakes. If like Perelman, I was certain of my proof, I would have found this a painfully under-whelming conclusion.

Perelman expected to feel proud, but instead he probably felt unappreciated and attacked. Instead of the parade he may have hoped for, he had to wait for a long time, only to face disappointment and frustration. This reminds me of an old joke:

A genie is trapped in a lantern at the bottom of the sea. He vows, “I will give one million dollars to the person who frees me.” One thousand years pass. He changes his vow, “I will give any amount of money to the one who frees me.” Another thousand years pass. He ups the ante, “I will give any amount of money and two more wishes to the person who frees me.” Another thousand years pass. He promises, “I will kill the one who frees me.”

Third explanation. Perelman was profoundly disappointed in the math community. Unlike the genie, Perelman didn’t want to kill anyone, but he did want to express his disillusionment. Perhaps that is why he rejected a million dollars.

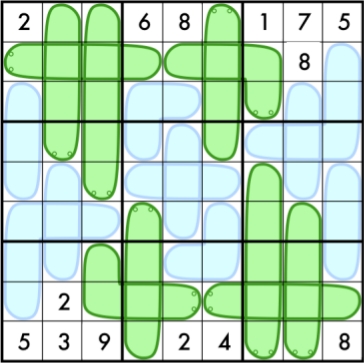
Do you like challenging puzzles? Are you tired of sudoku? Here’s your chance to try your hand at puzzles that are designed for world puzzle championships.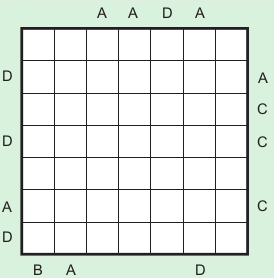

I’ve already done the homework for you — and it turned out to be more complicated than I anticipated. The world puzzle federation has a website, but unfortunately they are lazy or secretive. It is difficult to find puzzles there. A few puzzles are available in the World Puzzle Federation Newsletters.

Since I am stubborn, I spent a lot of time looking for championship puzzles. I found them in books. Here is the list I compiled so far. If you too are interested in high-level puzzles, this ought to make your search a lot easier. The book titles are confusing, so I added a description of what’s in them.

• Games Magazine Presents Brain Twisters from the First World Puzzle Championships — Warm-up puzzles, US/Canada Qualifying test, First World Championship, Foreign team puzzles.
• Games Magazine Presents Brain Twisters from the World Puzzle Championships, Volume 2 — US/Canada Qualifying test, Second World Puzzle Championship, Third World Puzzle Championship.
• Games Magazine Presents Brain Twisters from the World Puzzle Championships, Volume 3 — Fourth World Puzzle Championship, Fifth World Puzzle Championship.
• World Puzzle Championships Omnibus, Volume 1 — Contains World-Class Puzzles from the World Puzzle Championships Volumes 1-3 below.
• World-Class Puzzles from the World Puzzle Championships, Volume 1 — Sixth World Puzzle Championship and Seventh World Puzzle Championship.
• World-Class Puzzles from the World Puzzle Championships, Volume 2 — 1999 Qualifying test, Eighth World Puzzle Championship.
• The New York Times Sunday Crossword Omnibus, Volume 3 — 2000 Qualifying test, Ninth World Puzzle Championship.
• World-Class Puzzles from the World Puzzle Championships, Volume 4 — 2001 Qualifying test, Tenth World Puzzle Championship.
• World-Class Puzzles from the World Puzzle Championships, Volume 5 — 2002 Qualifying test, Eleventh World Puzzle Championship.
• Random House World-Class Puzzles — 2002 Qualifying test, Twelfth World Puzzle Championship. (The year for the qualifying test is probably a typo as puzzles differ from the qualifying test in the previous book,)

One of my favorite puzzle types is Easy as ABC. You have to fill one of A, B, C, and D in each row and column. The letters outside the grid indicate which letter you see first from that direction. Here is one from the 2011 newsletter:

* * *

Engraved on a mathematician’s tombstone: “Q.E.D.”

* * *

—You act very brave on the Internet. But could you repeat this looking into my eyes?
—Sure. Send me your picture.

* * *

—Your birthday?
—December 26^th.
—What year?
—Every year.

* * *

Teacher: “How much do we get if we cut eight into two halves?”
Student: “Two threes, if we cut vertically; and two zeros, if we cut horizontally.”

Two girls. One is older and more experienced. The other is younger and more naive. Which of these two girls will the unnamed male narrator choose? What a great plot for a math book.

I am talking about Hiroshi Yuki’s book Math Girls. The plot allows the author to discuss math on different levels. Miruka’s math is more advanced and mysterious. Tetra’s math is simpler and more transparent.

The book starts discussing sequences and patterns. Can you guess the pattern behind the sequence: 1, 2, 3, 4, 6, 9, 8, 12, 18, 27, …? Can you explain how the beginning of this sequence might be very deceptive?

For the answer, you can read the book, which also discusses tons of fun topics: prime numbers, sum of divisors, absolute values, rotations and oscillations, De Moivre’s formula, generating functions, arithmetic and geometric means, differential and difference operators, Catalan numbers, infinite series, harmonic numbers, zeta function, Taylor series, partitions, and more.

I usually do not like math fiction, but this is more math than fiction. It’s quite superior to most other math books I’ve read, for it shows the unity of mathematics. It allows the readers to discover connections among different parts of mathematics, and it accomplishes this in a very thrilling way. Frankly, more thrilling than the romantic sections.

The fictional element brings an additional value to the book. The author uses dialogue to discuss points that are usually skipped in regular text books. The two girls give the narrator an opportunity to explore math on different levels: to talk about heavy stuff with Miruka and to provide explanations with Tetra.

I expected to be more interested in the sections dealing with advanced math. But the book is so well-written that the simpler things were a lot of fun, too. For example, I never before noticed that the column notation for n choose k is exactly the same as for a 2d vector with coordinates n and k. And I will never ever shout “zero” because the exclamation makes it “one”.

I have a problem with my binocular vision. The muscles that are responsible for moving my eyes outwards are very weak, much weaker than the muscles that move my eyes inwards. When I am very tired, I can’t focus on people or things that are far away. I start seeing doubled monsters with extra eyes and noses.

Luckily, instead of looking scary, the monsters look familiar. In fact, they look exactly like Picasso’s portraits. I bet Picasso had problems with his eye muscles.

More than ten years ago I went through a process of psychotherapy which, although very painful, was extremely successful. When I tell my friends about this, they are interested in knowing what can be gained through psychotherapy, so here’s my story.

I was living in Princeton, NJ, and I was very tired all the time. My primary care doctor told me that I was depressed and needed to do psychotherapy. A friend of mine recommended Dr. Ella Friedman. During my first visit Ella told me that I block my negative emotions. I protested. All my life I truly tried to be honest with myself. She insisted. I had nothing to lose because I had to solve the problem of my constant exhaustion and I had no other potential solutions. Besides, I liked her very much. So I decided to play along and started my search looking for negative emotions.

For some time I tried to convince Ella that if my best friend broke my favorite mug I wouldn’t get angry with her. Ella tried to convince me otherwise. She pushed me back in time to the source of my beliefs and feelings. After several months of therapy, I discovered that I had a strong underlying belief that for my mother to love me, I must be a good girl who is always fair. Since my friend who broke the mug didn’t do it on purpose, I wasn’t allowed to be angry with her. I repressed all my angry feelings.

It took a lot of time for Dr. Friedman to rewire me and persuade me that my negative emotions do not mean that I am a bad girl. My actions define my goodness, not my emotions. I resisted. She had already convinced me that I might have negative emotions, but I didn’t want to look at them. The power forcing me to block my emotions was the threat that my mother would withdraw her love if I wasn’t a good girl. Dr. Friedman converted me. I started to believe her and continued more vigorously searching for my hidden emotions. Finally one day I collapsed in the shower. I actually felt my blocked emotions flooding me.

Negative emotions protect us. If someone treats you badly you need to be able to recognize it and get away from the danger. Because I didn’t see my emotions I stayed in situations, like toxic relationships, that caused me great pain, without realizing it.

My psychotherapy didn’t stop then. We started working on how to understand my emotions and how to process them. Now when someone is talking to me, I listen not only with my ears, but also with my gut. Suppose someone tells me, “I am so glad to see you,” but I feel a strange tightness in my stomach. I start wondering what the tightness is about, and usually can figure it out. For the first time I was able to hear my gut and it was more illuminating than what I was hearing with my ears. All my life I processed information as text. Now the sentence “I am so glad to see you” has many different meanings.

The therapy changed my life. It feels as if I added a new sense to my palette  of senses. I feel as if I was color blind for many years and at last I can see every color. Now that I’ve learned to recognize my pain, I can do something about it. I am so much happier today than I ever was before. While my friends may not have consciously recognized the big change in me, they have stopped calling me clueless and now often come to me for advice.

Did this solve my problem of tiredness? When Ella Friedman told me that I was no longer depressed, I still felt tired. I started investigating it further. It turns out that the depression was a result of the tiredness, not the other way around. It seems that I have a sleeping disorder and an iron problem.

SEAHOP created a practice puzzle, called “Making Connections,” that includes me. It seems I am making connections.