Tanya Khovanova's Math Blog

In my days of competing in math, I met guys who could solve any geometry problem by using coordinates: first they would assign variables to represent coordinates of different points, then they would write and solve a set of equations. It seemed so boring. Besides, this approach doesn’t provide us with any new insight into geometry.

I find geometric solutions to geometry problems much more interesting than algebraic solutions. The geometric solutions that use geometric transformations are often the shortest and the most beautiful.

I.M. Yaglom wrote a great trilogy called The Geometric Transformations. The first book of this trilogy discusses translations, rotations and reflections. The second one — looks at similarity transformations, and the third one talks about affine and projective transformations. A lot of beautiful problems with their solutions are scattered throughout these books. They include all my favorite problems related to transformations.

I think geometry is the weakest link for the USA math team. So we have to borrow the best geometry books from other countries. This trilogy was translated from Russian and Russians are known for their strong tradition of excellence in teaching geometry.

Below you can find sample problems from Geometric Transformations 1, Geometric Transformations 2 and Geometric Transformations 3 — not necessarily in this order.

I.M. Yaglom wrote a great trilogy called The Geometric Transformations. The first book of this trilogy discusses translations, rotations and reflections. The second one — looks at similarity transformations, and the third one talks about affine and projective transformations. A lot of beautiful problems with their solutions are scattered throughout these books. They include all my favorite problems related to transformations.

I think geometry is the weakest link for the USA math team. So we have to borrow the best geometry books from other countries. This trilogy was translated from Russian and Russians are known for their strong tradition of excellence in teaching geometry.

Below you can find sample problems from Geometric Transformations 1, Geometric Transformations 2 and Geometric Transformations 3 — not necessarily in this order.

Problem 1. Let A be a point outside a circle S. Using only a straightedge, draw the tangents from A to S.

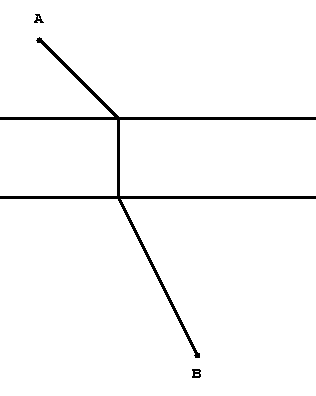
Problem 2. At what point should a bridge be built across a river separating two towns A and B (see figure) in order that the path connecting the towns be as short as possible? The banks of the river are assumed to be parallel straight lines, and the bridge is assumed to be perpendicular to the river.

Problem 3. Suppose you have two lines drawn on a piece of paper. The intersection point A of the two lines is unreachable: it is outside the paper. Using a ruler and a compass, draw a line through a given point M such that, were the paper bigger, point A would belong to the continuation of the line.

In the book “Mythematics: Solving the Twelve Labors of Hercules” Michael Huber adds details to Hercules’ labors so that in order that he can do each task, you need to help Hercules solve two or three math problems. For example, to defeat the Nemean Lion Hercules needs to solve the problem “Zeus Makes a Deal”, which is a Greek-myth version of the Monty Hall problem.

The problems in Mythematics are quite advanced. They range in topic from algebra, geometry and probability to differential equations and integral calculus. Plus, as a reward for helping Hercules, Huber gives you variations on Sudoku puzzles.

Solving some nice math problems might not be the only reason for people to buy this book. Here are some other reasons:

• Greek myth lovers may find extra motivation to do mathematics.
• People will earn that extra gratification of imagining that they are doing good deeds while solving math puzzles.
• Puzzle lovers can learn or refresh their knowledge of Hercules’ labors.

I like Huber’s approach. Future possibilities for more books are endless. Let’s write new math problems based on Harry Potter, Batman, the Bible or, maybe, The Joy of Sex.

In the book “Mythematics: Solving the Twelve Labors of Hercules” Michael Huber adds details to Hercules’ labors so that in order that he can do each task, you need to help Hercules solve two or three math problems. For example, to defeat the Nemean Lion Hercules needs to solve the problem “Zeus Makes a Deal”, which is a Greek-myth version of the Monty Hall problem.

The problems in Mythematics are quite advanced. They range in topic from algebra, geometry and probability to differential equations and integral calculus. Plus, as a reward for helping Hercules, Huber gives you variations on Sudoku puzzles.

Solving some nice math problems might not be the only reason for people to buy this book. Here are some other reasons:

• Greek myth lovers may find extra motivation to do mathematics.
• People will earn that extra gratification of imagining that they are doing good deeds while solving math puzzles.
• Puzzle lovers can learn or refresh their knowledge of Hercules’ labors.

I like Huber’s approach. Future possibilities for more books are endless. Let’s write new math problems based on Harry Potter, Batman, the Bible or, maybe, The Joy of Sex.

I bought the book “Heard on The Street: Quantitative Questions from Wall Street Job Interviews” by Timothy Falcon Crack several years ago when I was looking for a job and felt that working in finance was a possibility. Despite having bought it simply to prepare for employment interviews, I actually enjoyed the math problems in the book.

The book has problems in logic, probability, statistics and finance, as well as a very useful chapter of general interview questions. If you’re interested in buying this book, I should mention that some questions require calculus and knowledge of financial terms.

I love the author’s taste in problems, and here are some sample questions from the book.

Question 2.7: How many degrees (if any) are there in the angle between the hour and minute hands of a clock when the time is a quarter past three?

Question 5.1.14: Welcome to your interview. Sit in this chair. Excuse me while I tie your arms and legs to the chair. Thank you. Now we are going to play “Russian roulette.” I have a revolver with six empty chambers. Watch me as I load the weapon with two contiguous rounds (i.e., two bullets side-by-side in the cylindrical barrel). Watch me as I spin the barrel. I am putting the gun against your head. Close your eyes while I pull the trigger. This is your lucky day: you are still alive! Our game differs from regular Russian roulette because I am not going to add any bullets to the barrel before we continue, and I am not going to give you the gun.
My question for you: I am going to shoot at you once more before we talk about your résumé. Do you want me to spin the barrel once more, or should I just shoot?

Question 6.1.16: Tell me something you tried but ended up quitting on.

I can tell you what I would have answered to the last question: I tried smoking, but ended up quitting.

I bought the book “Math Doesn’t Suck: How to Survive Middle School Math Without Losing Your Mind or Breaking a Nail“ by Danica McKellar because I couldn’t resist the title. Sometimes this book reads like a fashion magazine for girls: celebrities, shopping, diet, love, shoes, boyfriends. At the same time it covers elementary math: fractions, percents and word problems.

You can apply math to anything in life. Certainly you can apply it to fashion and shoes. I liked the parallel between shoes and fractions that Danica used. She compared improper fractions to tennis shoes and mixed numbers to high heels. It is much easier to work with improper fractions, but mixed numbers are far more presentable.

Danica is trying to break the stereotype that girls are not good at math by feeding all the other stereotypes about girls. If you are a typical American girl who hates math and missed some math basics, this book is for you. If you want to discover whether the stars are on your side when you are learning math, the book even includes a math horoscope.

I got a funny book for a gift called Plato and a Platypus Walk into a Bar…: Understanding Philosophy Through Jokes. I couldn’t stop reading it. This book is an overview, and thus not very deep, but I enjoyed being reminded of philosophical concepts I’ve long since forgotten. Besides, I collect math jokes and many philosophical jokes qualify as mathy ones.

For example, self-referencing jokes:

Relativity — this term means different things to different people.

I especially liked jokes related to logic:

If a man tries to fail and succeeds, which did he do?

I knew most of the jokes, but here’s a math joke I never heard before:

Salesman: “Ma’am, this vacuum cleaner will cut your work in half.”
Customer: “Terrific! Give me two of them.”

The Symmetries of Things by John H. Conway, Heidi Burgiel, and Chaim Goodman-Strauss is out. It is a beautiful edition with great pictures.

The first chapter is very nicely written and might be recommended to high school and undergraduate students. It covers symmetries of finite and infinite 2D objects.

The second chapter adds color to the theory. For beautiful colorful pictures with symmetry, there are two symmetry groups: the group that preserves the picture while ignoring its coloring and the group that preserves the picture while respecting its coloring. The latter group is a subgroup of a former group. This second chapter discusses all possible ways to symmetrically color a symmetric 2D picture. The chapter then continues with a discussion of group theory. This chapter is much more difficult to read than the first chapter, as it uses a lot of notations. The pictures are still beautiful, though.

The third chapter is even more difficult to read and the notations become even heavier. This chapter discusses hyperbolic groups and symmetries of objects in the hyperbolic space. Then the chapter moves into 3D and 4D. I guess that some parts of the second and the third chapters are not meant for light reading; they should be considered more as reference materials.

Here are pictures of a musnub cube (multiplied snub cube), built by John H. Conway. It is an infinite Archimedean polyhedron. The description of it is on page 338 and the diagram is on page 339 of the book. This object was glued together from stars and squares. Each corner of the square is glued to a point of one star and to an inside corner of another star. Mathematically, a star is not a regular polygon. If you look at stars with your mathematical eye, each star in the picture is not just a star, but rather the union of a regular hexagon with 6 regular triangles. That means the list of the face sizes around each vertex of a musnub cube officially is represented as 6.3.4.3.3.

The second picture shows a finished musnub cube. You can’t really finish building an infinite structure. Right? It is finished in the sense that John Conway finished doing what he was planning to do: to construct a part of a musnub cube inside a regular triangular pyramid.

Did I mention that I like the pictures in The Symmetries of Things?