Tanya Khovanova's Math Blog

I was teaching my students PIE, the Principle of Inclusion and Exclusion. This was the last lesson of 2010 and it seemed natural to have a party and bring some pie. It appears that the school has a new rule. If I want to bring any food to class, I need to submit a request that includes all food ingredients. The administrators send it to the parents asking them to sign a permission slip and then, if I receive all the slips back in time, I can bring pie to school. We had to study PIE without pie.

Our most important task as parents and teachers is to teach kids to make their own decisions. They are in high school; they know by now about their own allergies and diets; they should be able to avoid foods that might do them harm. I understand why schools create such rules, but we are treating the students like small children. We can’t protect them forever; they need to learn to protect themselves.

Next semester, we will study the mathematics of fair division. I will have to teach them how to cut a cake without a cake.

I gave my students a problem from the 2002 AMC 10-A:

Tina randomly selects two distinct numbers from the set {1, 2, 3, 4, 5}, and Sergio randomly selects a number from the set {1, 2, …, 10}. The probability that Sergio’s number is larger than the sum of the two numbers chosen by Tina is: (A) 2/5, (B) 9/20, (C) 1/2, (D) 11/20, (E) 24/25.

Here is a solution that some of my students suggested:

On average Tina gets 6. The probability that Sergio gets more than 6 is 2/5.

This is a flawed solution with the right answer. Time and again I meet a problem at a competition where incorrect reasoning produces the right answer and is much faster, putting students who understand the problem at a disadvantage. This is a design flaw. The designers of multiple-choice problems should anticipate mistaken solutions such as the one above. A good designer would create a problem such that a mistaken solution leads to a wrong answer — one which has been included in the list of choices. Thus, a wrong solution would be punished rather than rewarded.

Readers: here are three challenges. First, to ponder what is the right solution. Second, to change parameters slightly so that the solution above doesn’t work. And lastly, the most interesting challenge is to explain why the solution above yielded the correct result.

I am coaching my AMSA students for math competitions. Recently, I gave them the following problem from the 1964 MAML:

The difference of the squares of two odd numbers is always divisible by:
A) 3, B) 5, C) 6, D) 7, E) 8?

The fastest way to solve this problem is to check an example. If we choose 1 and 3 as two odd numbers, we see that the difference of their squares is 8, so the answer must be E. Unfortunately this solution doesn’t provide any useful insight; it is just a trivial calculation.

If we remove the choices, the problem immediately becomes more interesting. We can again plug in numbers 1 and 3 to see that the answer must be a factor of 8. But to really solve the problem, we need to do some reasoning. Suppose 2k + 1 is an odd integer. Its square can be written in the form 4n(n+1) + 1, from which you can see that every odd square has remainder 1 when divided by 8. A solution like this is a more profitable investment of your time. You understand what is going on. You master a method for solving many problems of this type. As a bonus, if students remember the conclusion, they can solve the competition problem above instantaneously.

This is why when I am teaching I often remove multiple choices from problems. To solve them, rough estimates and plugging numbers are not enough. To solve the problems the students really need to understand them. Frankly, some of the problems remain boring even if we remove the multiple choices, like this one from the 2009 AMC 10.

One can holds 12 ounces of soda. What is the minimum number of cans needed to provide a gallon (128 ounces) of soda?

It’s a shame that many math competitions do not reward deep analysis and big-picture understanding. They emphasize speed and accuracy. In such cases, plugging in numbers and rough estimates are useful skills, as I pointed out in my essay Solving Problems with Choices.

In addition, smart guessing can boost the score, but I already wrote about that, too, in How to Boost Your Guessing Accuracy During Tests and To Guess or Not to Guess?, as well as Metasolving AMC 8.

As the AMC 10 fast approaches, I am bracing myself for the necessity to include multiple choices once again, thereby training my students in mindless arithmetic.

Many people ask me when is a good time to teach kids math. In my experience, it can never be too early. You just need to keep some order. Multiplication should be taught after addition, and negative numbers after subtraction. Kids should remember multiplication by heart at the age of seven. They can understand negative numbers as early as four.

In the picture I am explaining Platonic solids to four-month-old Eli, the son of my friends. His homework is to chew on a dodecahedron.

I am starting yet another part-time job as the Head Mentor at PRIMES, a new MIT research program for high schoolers. I am very excited about this program, for it will be valuable not only to kids who want to become researchers, but also to kids who just want to see what research is like. Kids who want to learn to think in a new way will also find it highly useful.

PRIMES is in many ways similar to RSI, which it augments and complements. There are also a lot of differences. Keep in mind that I am only comparing PRIMES to the math part of RSI, with which I was working as a coordinator for two years. I do not know how RSI handles other sciences.

Different time scale. RSI lasts six weeks; PRIMES will take about a year. I already wrote about some peoples’ skepticism towards RSI in my piece called “Fast Food Research?.” PRIMES creates a more natural pace for research.

Choices. Because of the time schedule at RSI, students get their project as soon as they start. Students who realize by the end of the second week that they do not like their project are at a disadvantage: if they do not change their project, they’re stuck with something that does not inspire them or is too difficult, and if they do change their project, they won’t have enough time to do a great job. At PRIMES students will have time to talk to the mentors before starting their project, so that they can participate in choosing their project. Depending on how it goes later, they’ll have time to try several different directions. I believe that the best research comes from the heart: students who have the time and opportunity to shape their choices will be more invested in their project.

Application process. At RSI, The Center for Excellence in Education reviews the applications. Even though they usually do a superb job at sending us great students, I believe it would be an advantage if mentors were able to influence the review process, for they might find even better matches to their projects. At PRIMES, the mentors will have this opportunity to review the applications.

Geography. RSI accepts students from all over the US and from some other countries. PRIMES can only accept local students — those who live close enough to visit MIT once a week for four months. Because of this restriction, PRIMES is recruiting from a smaller pool of students than RSI. But for local students it means that it will be easier to get accepted to PRIMES than to RSI.

Coaching. At RSI, students get a lot of coaching. I think that every student is in close contact with four adults. Two of them are from the math department — mentor and coordinator (that’s me!) — and two tutors from CEE. PRIMES will have less coaching. A student will have a mentor and me, the head mentor. In addition, mentors might arrange for students to talk to the professors who originated their projects.

Immersion. RSI students are physically present. They are housed at MIT with the expectation that they completely devote their time to their research. Students at PRIMES will be integrating their research into the rest of their lives and their commitments. That will require good organizational skills and a lot of self-discipline. RSI students have discipline imposed on them by their situation — which may be an advantage to them.

Olympiads. While they are at RSI, students can’t go to IMO or other summer activities. This is why many strong Olympiad students choose not to go to RSI, or they turn down an RSI acceptance if in the meantime they have gotten on to an Olympic team. At PRIMES you can do both. It is possible to go to an Olympiad, in addition to writing a paper.

Grade. RSI students have to be juniors. There are no grade limitations for PRIMES. Thus, it is possible to go to PRIMES in one’s senior year. In this case, it may be too late to use PRIMES on college applications, but it is perfectly fine for the sake of research itself. Or it might be possible to go to PRIMES as a sophomore, and then apply for RSI the next year. This will strengthen the student’s application for RSI.

RSI is well-established and has proven itself. PRIMES is new and hopefully will offer young mathematicians additional opportunities to try research.

I think that the American system of education creates a lot of pressure for teachers to drill their students for standardized tests and multiple choice questions. This blocks creative thinking. Every program like PRIMES is very good for unleashing students’ creativity and contributing to the development of the future thinkers of American society.

by David Wilson

I was somewhat taken aback by the popularity of my earlier essay “Divisibility by 7 is a Walk on a Graph.” Tanya tells me it got a good number of hits. The graph in that article is rather crude, and takes a bit of care to use, because the arrows go off in random directions from each node. So taking a hint from a commenter on the first graph, I redrew the graph, sacrificing planarity in favor of ease of use. Specifically, I arranged the black arrows in a counterclockwise circle, which makes them easy to follow.

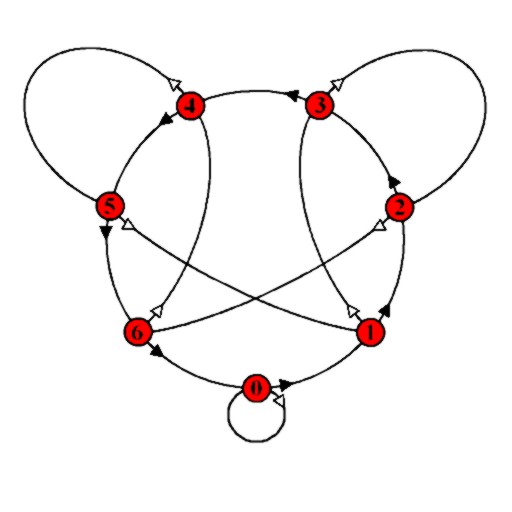The graph is used in the same way as the first graph. To find the remainder on dividing a number by 7, start at node 0, for each digit D of the number, move along D black arrows (for digit 0 do not move at all), and as you pass from one digit to the next, move along a single white arrow.

For example, let n = 325. Start at node 0, move along 3 black arrows (to node 3), then 1 white arrow (to node 2), then 2 black arrows (to node 4), then 1 white arrow (to node 5), and finally 5 black arrows (to node 3). Finishing at node 3 shows that the remainder on dividing 325 by 7 is 3.

I fancy it to be a little animal face.

Here is a fun math activity I use with my students, after I teach them to play the game of set. To other teachers — feel free to clone this idea.

First, I ask the students if they know what a magic square is. They usually do know that a magic square is a three-by-three square of distinct digits, so that every row, column and diagonal has the same sum. Then I ask them what a magic set square might be. Often they guess correctly that it is a three-by-three square made of set cards, so that every row, column and diagonal form a set. Once that’s established, I have them build magic set squares.

While they’re building them, I ask a lot of questions, from how many cards there should be in the deck to how many different sets there are.

Once the squares are built, I ask them what a magic set cube might be. Their next task is to use their magic set squares as the bottom layer in building magic set cubes. In order to see all the cards in the cube, I instruct them to arrange the layers (bottom, middle and top) side-by-side.

As they’re working on their cubes, I continue quizzing them. How many main diagonals does a cube have? Once they confirm that the answer is four, I ask them to show me those diagonals in their magic set cubes and check that they are sets. I might also ask them how many different magic set squares should be inside a magic cube. This is a theoretical math question they need to answer before finding them in their own model. Next they need to identify the different sets that form lines inside their cubes.

At this point, some students guess my next request: to construct a magic set hypercube.

After students build their hypercubes, they never want to destroy them. They like comparing the different hypercubes and often take photos of them. If there’s still time left, I can continue in several directions. For example, they can count the main diagonals of the hypercube and find them in their models. Alternatively, they can find a “no set” — the largest possible set of cards inside a magic set hypercube that doesn’t contain a set.

Math is usually about thinking, but this is one activity the students can do with their hands. And that adds another layer of magic.

By Tanya Khovanova and Richard Stanley

This essay is written especially for high school and undergrad math lovers who enjoy problem solving and who plan to major in mathematics. One of the authors, Tanya, often received this advice when she was an undergraduate in Russia: “Problem solving is child’s play. You’ll have to change your attitude if you plan to succeed in research.”

Perhaps that’s why some famous problem solvers, even those who won gold medals at IMO, became not-so-famous mathematicians. To help you avoid that fate, we’ll discuss the ways in which research is unlike problem solving.

Is research different from problem solving?

Yes and no. There are many mathematicians who continue problem solving as their form of research. Remember Paul Erdos who used to suggest a lot of problems and even offered money rewards for solutions. Many mathematicians solve problems posed by other people. You might consider Andrew Wiles as the ultimate math problem solver: he proved Fermat’s last theorem, which had been open for 400 years. Though he could not have done it without the many theories that had already been generated in the search to find the elusive proof.

You can become a mathematician and continue to look around you for problems to solve. Even though this is still problem solving, the problems will be very different from competition problems, and you will still need to adjust to this type of research.

Problems you solve during research

So, what is the difference between problems that mathematicians solve during competition and the problems they tackle for their research?

Expected answer. In competition problem solving you know there is a solution. Often you know the answer, but you just need to prove it. In research there is no guarantee. You do not know which way it will go. For this reason finding counter-examples and proving that some ideas are wrong is a positive contribution, for it can eliminate some possibilities. So one adjustment is that you might start valuing negative answers.

Difficulty level. Competition problems are designed to be solved in one hour, so you are expected to generate an idea in just minutes. In research the problem might drag on for years, because it is far more difficult. If you get used to the instant gratification of competition problem solving, you might find the lengthy work of research frustrating. It’s very important to adjust your expectations so that you won’t drop a problem prematurely. You need to measure progress in small intermediate steps and learn to appreciate this different rhythm.

Motivation. Although you miss the euphoria of finding quick solutions, you get a different kind of reward with research. Because no one knows the answer in advance, when you solve the problem, you are the first to do so. You have opened up a new truth.

Time limits. In competitions you have a time limit for every problem. In research you set your time limits yourself. That allows you to put a problem aside and come back later if necessary. In a sense you can think about several problems at the same time.

Your passion. You can choose your problems yourself. Research is much more rewarding if you follow your heart. In competitions you have to spend time on problems you might not like. Here you have an option to choose and pick only the problems that appeal to you. Thus, you become more motivated and as a result more successful.

Finding a problem

After solving problems posed by other people, the next step is to pose math problems yourself. As we mentioned before, in research you do not always have a strictly-defined problem. It is a significant adjustment to move from solving already-defined problems to posing the problems yourself.

Generalizations. Often you can generalize from an existing problem to more general cases. For example, if you see a problem for n=3, you can wonder what happens for any n, or for any prime n.

Being on the lookout. Sometimes a situation puzzles you, but you can’t formulate a specific problem around that situation. For example, why do most of the terms in the sequence end in 9? Is there a reason for that? Or, you might find that a formula from your integrable systems seminar is similar to a formula from your representation theory class. This might lead you to the essential research question: “What is going on?” You always need to be on the lookout for the right questions.

Value. When you create your own research problems it is crucial to always ask yourself: Is the problem I am creating important? What is the value of this problem? There is no a good reason to create random generalizations of random problems. If the problem you found interests you very much, that is the first sign that it might interest other people; nonetheless, you should still ask yourself how this problem will help advance mathematics.

Mathematics is not only problem solving

There are other things to do than solve problems. There are many mathematicians who work differently, who don’t solve problems or don’t only solve problems. Here are some of the many options mathematicians have:

Building structures. You may not be interested in calculating the answer to a question, but rather in building a new structure or a new theory.

Advancing the language. When you invent new definitions and new notations, you will help to simplify a math language so that the new language will allow you to prove your results and other peoples’ results faster and clearer.

Unification. Sometimes you notice two results in two different areas of mathematics with some kind of similarity. Explaining why these results are the same might create a new understanding of things. It is great to unify two different areas of mathematics.

Explaining. Very often proofs are not enough. Why is something true? What’s the reason and what’s the explanation? It is good to ask yourself a “why” question from time to time, such as, “Why is this proof working?” When you find an answer, it might become easier to understand what to do next and how to generalize your proof.

Directions. Many mathematicians are valued not for the problems they solve or suggest, but for ideas and directions they propose. Finding a new direction for research can generate unexpected opportunities and create tons of math problems on the way. It can be valuable to come up with good conjectures, even if you have no hope of solving them yourself. Two example of this are the Weil conjectures (eventually proved by Deligne) and the Langlands program, which is still incomplete but which has generated a huge amount of important research.

Vision. What is the most general thing that can be proved by this technique? What kinds of improvements and refinements are there? It is good to step back from the problem you solved and meta-think about it.

As you can see, problem solving is just the beginning of all that mathematics can offer you. Mathematicians find these other options very rewarding, so it’s worth your while to try these varied aspects of mathematical work to see if you have a taste for other things. If you don’t venture beyond problem solving you might miss the full beauty of mathematics.

My guest blogger is David Wilson, a fellow fan of sequences. It is a nice exercise to understand how this graph works. When you do, you will discover that you can use this graph to calculate the remainders of numbers modulo 7. Back to David Wilson:

I have attached a picture of a graph.

Write down a number n. Start at the small white node at the bottom of the graph. For each digit d in n, follow d black arrows in a succession, and as you move from one digit to the next, follow 1 white arrow.

For example, if n = 325, follow 3 black arrows, then 1 white arrow, then 2 black arrows, then 1 white arrow, and finally 5 black arrows.

If you end up back at the white node, n is divisible by 7.

Nothing earth-shattering, but I was pleased that the graph was planar.

My son, Sergei Bernstein, got accepted to MIT through early action. Because the financial costs of studying at MIT worried me, I insisted that Sergei also apply to Princeton and Harvard, as I had heard they give generous financial packages. In the end, Sergei was rejected by Princeton and wait-listed and finally rejected by Harvard. Though many people have been rejected by Princeton and Harvard, not too many of them have won places on US teams for two different international competitions — one in mathematics and the other in linguistics. To be fair, Sergei was accepted by these teams after Princeton had already rejected him. Nonetheless, Sergei has an impressive mathematical resume:

• In 2005 he was the National MathCounts Written Test Champion.
• In 2005 he was the National MathCounts Master’s Round Champion.
• In 2007 and 2009 he was a USAMO winner.
• In 2008 he passed Math 55a at Harvard taught by Dennis Gaitsgory, which is considered to be the hardest freshman math course in the country. More than 30 students started it and less than 10 finished. Sergei was one of the finishers, and he was only a high school junior.
• In 2007, 2008 and 2009 he competed at a 12th grade level at the Math Kangaroo, while he actually was in 10th, 11th and 12th grade. He placed first all three times.
• In 2009 he was on the US team at the Romanian Masters in Mathematics competition, which might be a harder competition than the International Mathematical Olympiad. He got a silver medal and was second on the US team.
• In 2009 he placed 5th in the North American Computational Linguistics Olympiad, making it to the Alternate US Team for the International Linguistics Olympiad.

I am trying to analyze why he was rejected and here are my thoughts.

1. His application forms to Harvard and Princeton were different from MIT. Yes, MIT was his first choice and he wrote a customized essay for MIT. For other places he had a common essay. But as he was supposed to be flagged as a top math student, his essay should have been irrelevant, in my opinion.
2. Admissions offices made a mistake. I can imagine that admissions offices never heard of the Romanian Masters in Mathematics competition, because it is a relatively new competition and the USA only joined it in 2009 for the first time. On its own, though, it should have sounded impressive. Also, they might not have known about the Math 55 course at Harvard, as usually high-schoolers do not take it. But that still leaves many other achievements. Many people told me that admissions offices know what they are doing, so I assume that I can disregard this point.
3. Princeton and Harvard knew that he wanted to go to MIT and didn’t want to spoil their admission rate. I do not know if colleges communicate with each other and whether Princeton and Harvard knew that he was admitted early to MIT. Because he had sent them a common application essay, they may have been suspicious that they weren’t his first choice.
4. Harvard and Princeton didn’t want him. I always heard that Harvard and Princeton want to have well-rounded people, whereas MIT likes geeks. I consider Sergei quite well-rounded as he has many other interests and achievements beyond mathematics. Perhaps his other accomplishments aren’t sufficiently impressive, making him less round than I thought he was.
5. Harvard and Princeton are not interested in mathematicians. Many people say that they want future world leaders. I think it is beneficial for a world leader to have a degree in math, but that’s just my personal opinion. And of course, to support their Putnam teams, it is enough to have one exceptional math student a year.
6. Sergei couldn’t pay. Yes, we marked on the application that we need financial help. In the current financial crisis it could be that even though Harvard and Princeton do not have enough money to support students, they do not want to go back and denounce their highly publicized generosity.

Many people told me of surprising decisions by Ivy League schools this year. The surprises were in both directions: students admitted to Ivy League colleges who didn’t feel they had much of a chance and students not admitted that had every right to expect a positive outcome. I should mention that I personally know some very deserving kids who were admitted.

I wonder if there has been a change in the financial demographics of the students Harvard and Princeton have accepted this year. If so, this will be reflected in the data very soon. We will be able to see if the average SAT scores of students go down relative to the population and previous years.

I do not know why Sergei wasn’t accepted; perhaps I’m missing something significant. But if it was because of our finances, it would be ironic: Sergei wasn’t admitted to Princeton and Harvard for the same reason he applied there.